Probabilistic modeling of the IGPM12 index

Authors

  • Samantha Gouvêa Oliveira PhD student in the Applied Statistics and Biometrics program - Department of Statistics (DET/UFV) https://orcid.org/0009-0003-8789-1597
  • Luciano Gonçalves Batista PhD student in the Applied Statistics and Biometrics program - Department of Statistics (DET/UFV)
  • Eduardo Campana Barbosa Professor at the Department of Statistics (DET/UFV)
  • Paulo César Emiliano Professor at the Department of Statistics (DET/UFV) https://orcid.org/0000-0002-1314-9002
  • Maurício Silva Lacerda Professor at the Teaching Support Department - Federal Institute of Education, Science and Technology of Rondônia (DAPE/IFRO) https://orcid.org/0000-0003-1209-3956
  • Kamila Andrade de Oliveira Professor at the Department of Agricultural Engineering, Campus Chapadinha (DEA/UFMA) https://orcid.org/0000-0002-6401-4132

Keywords:

Asymmetry, kurtosis, maximum likelihood

Abstract

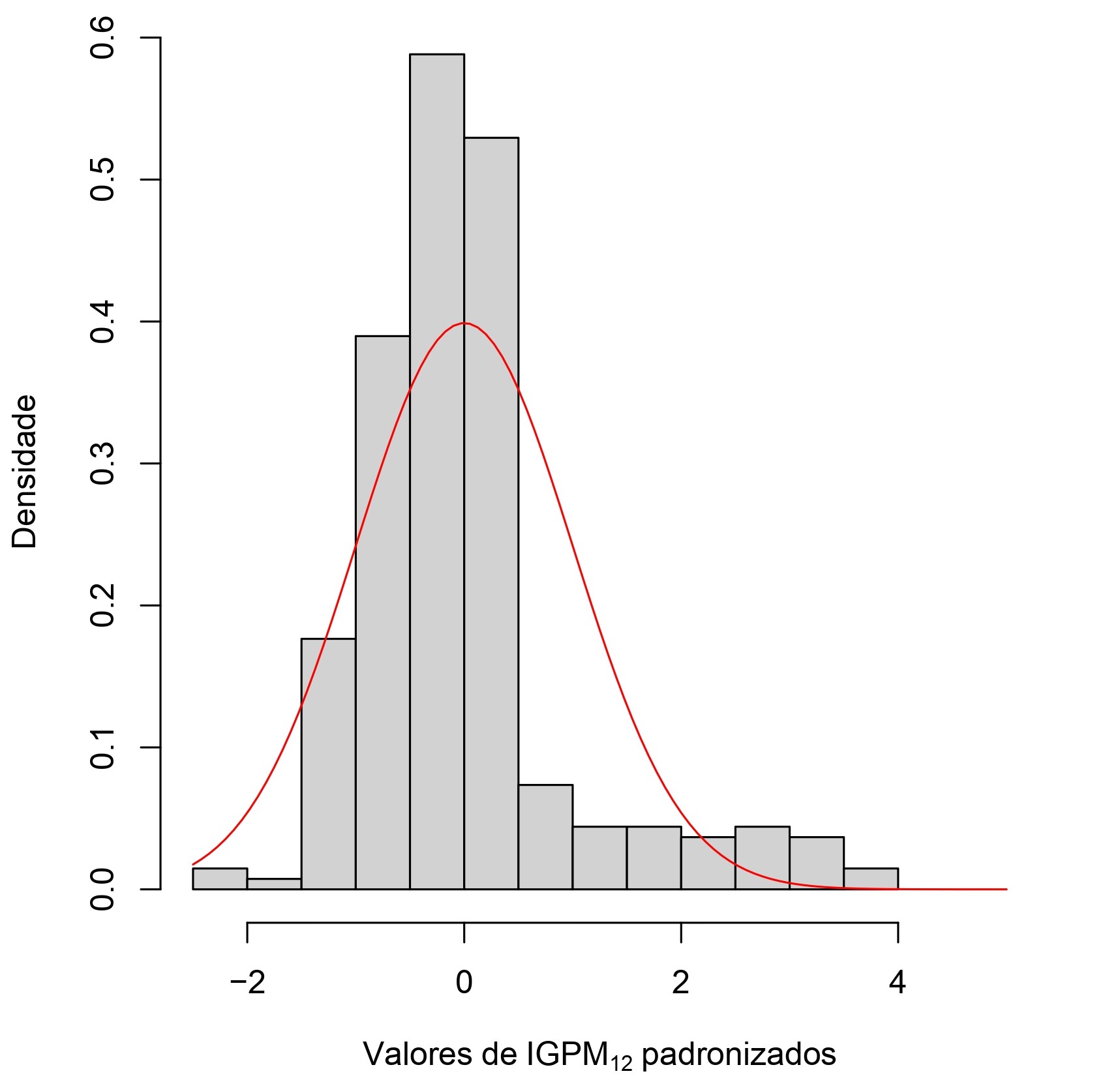
The objective of this work was to adjust a distribution to the IGPM12 data and estimate the probability of this index assuming certain values that may be specific to individuals, legal entities and investors who have commitments or financial investments whose investors are linked to the IGPM. To choose between the normal, Cauchy, logistic and LS t-Student distributions, the Kolmogorov-Smirnov test and the Akaike Information Criterion (AIC) were analyzed. The LS t Student model with parameters mean 7.29, standard deviation 3.99 and nu 2.20 was selected and with it the probabilities of interest were estimated. It is concluded that the most likely scenario (p = 62%) involves an adjustment of this index between 0% and 10% for the next 12 months.

References

AKAIKE, H. A new look at the statistical model identification. IEEE Transactions on

Automatic Control, Notre Dame, v. 19, n. 6, p. 717-723, 1974.

CONOVER, W. J. Practical Nonparametric Statistical. John Wiley & Sons Inc., New York,

p.

De SOUZA, J. A. Para entender os índices de preços: uma visão moderna. ˆ Revista de

Economia Mackenzie, v. 2, n. 2, 2004.

EMILIANO, P. C.; VIVANCO, M. J. F.; MENEZES, F. S. Information criteria: How do they

behave in different models?. Computational Statistics & Data Analysis, v. 69, p. 141-153,

LENTH, R. V. Algorithm AS 243 — Cumulative distribution function of the non-central t

distribution. Applied Statistics, v. 38, n. 185–189, 1989.

MARSAGLIA G., TSANG W. W., WANG J. Evaluating Kolmogorov’s distribution. Journal

of Statistical Software, v. 8, n. 18, 2003.

MATTOS, A. A infla ̧c ̃ao brasileira. Uma abordagem pratica e moderna antes e depois do

choque. Petr ́opolis: Vozes, 1986.

MILLER, R. B. Maximum likelihood estimation and inference: with examples in R, SAS, and

ADMB. 1. ed. [S.l.]: Wiley, 2011.

MOOD, A. M.; GRAYBILL, F. A.; BOES, D. C. Introduction to the theory of statistics.

Singapore: McGraw-Hill, 1974. 564 p.

R CORE TEAM. R: a language and environment for statistical computing. R Foundation for

Statistical Computing, Vienna, Austria. 2023.

Downloads

Published

01-06-2024

How to Cite

Oliveira, S. G., Batista, L. G., Barbosa, E. C., Emiliano, P. C., Lacerda, M. S., & Oliveira, K. A. de. (2024). Probabilistic modeling of the IGPM12 index. Sigmae, 13(2), 45–56. Retrieved from https://publicacoes.unifal-mg.edu.br/revistas/index.php/sigmae/article/view/2358

Issue

Vol. 13 No. 2 (2024): "Don't panic"

Section

Applied Statistics

License

Proposta de Política para Periódicos de Acesso Livre


Autores que publicam nesta revista concordam com os seguintes termos:

    1. Autores mantém os direitos autorais e concedem à revista o direito de primeira publicação, com o trabalho simultaneamente licenciado sob a Licença Creative Commons Attribution que permite o compartilhamento do trabalho com reconhecimento da autoria e publicação inicial nesta revista.

    1. Autores têm autorização para assumir contratos adicionais separadamente, para distribuição não-exclusiva da versão do trabalho publicada nesta revista (ex.: publicar em repositório institucional ou como capítulo de livro), com reconhecimento de autoria e publicação inicial nesta revista.

  1. Autores têm permissão e são estimulados a publicar e distribuir seu trabalho online (ex.: em repositórios institucionais ou na sua página pessoal) a qualquer ponto antes ou durante o processo editorial, já que isso pode gerar alterações produtivas, bem como aumentar o impacto e a citação do trabalho publicado (Veja O Efeito do Acesso Livre).